Find the short side of a triangle given a hypotenuse of 10 units Find the hypotenuse given a long side of 6 units Triangles are classified as "special right triangles" They are special because of special relationships among the triangle legs that allow one to easily arrive at the length of the sides with exact answers instead of decimal approximations when using trigFind the remaining sides of a 30°–60°90° triangle if 39 The shortest side is 1 40 "The shortest side is 3 41 The longest side is 8 42 The longest side is 5 43 The longest side is 44 'The longest side is 24 45 The medium side is 3V3 46 "The medium side is 2V3 47 The medium side is 6 48 The medium side is 4How To Find Sides Of A 30 60 90 Triangle 30 60 90 Triangle Therom Slidedocnow 30 60 90 Triangle Theorem Ratio And Formula Video Triangles On Sat Math Geometry Strategies And Practice How To Find The Sides Of A 30 60 90 Right Triangle Math Understanding 30 60 90 Triangles High School Math 30 60 90 Triangles Kates Math Lessons An Appearance Of A 30

The 30 60 90 Triangle Topics In Trigonometry
Find the sides of a 30 60 90 triangle
Find the sides of a 30 60 90 triangle-Find the remaining sides of a 30° – 60° – 90° triangle if the longest side is 9 The side opposite 60° is and the shortest side is ;Special Triangles Isosceles and Calculator This calculator performs either of 2 items 1) If you are given a right triangle, the calculator will determine the missing 2 sides Enter the side that is known After this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right triangle




30 60 90 Formula Learn Formula For Calculating The 30 60 90 Measures
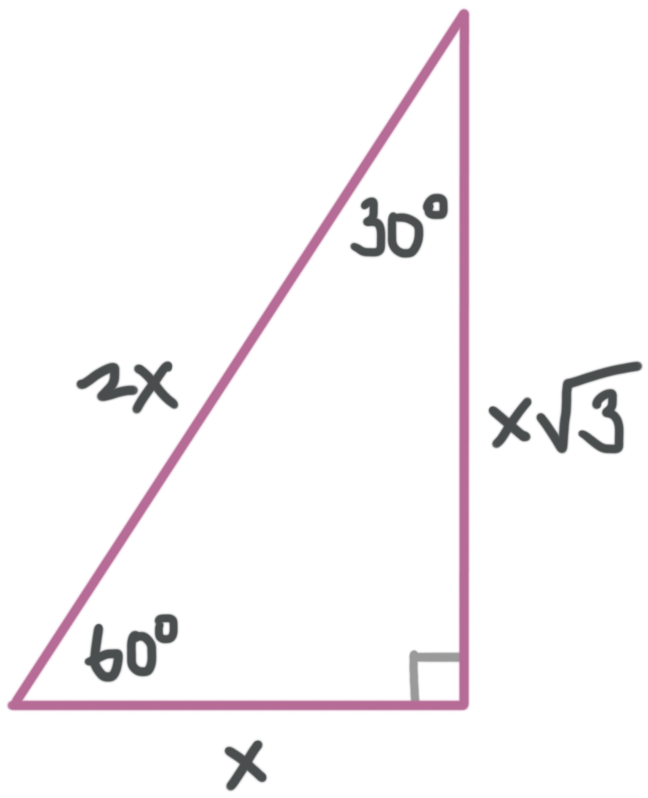
30 60 90 triangle sides If we know the shorter leg length a, we can find out that b = a√3 c = 2a If the longer leg length b is the one parameter given, then a = b√3/3 c = 2b√3/3 For hypotenuse c known, the legs formulas look as follows a = c/2 All degree triangles have sides with the same basic ratio Two of the most common right triangles are and degree triangles If you look at the 30–60–90degree triangle in radians, it translates to the following In any triangle, you see the following The shortest leg is across from the 30degree angle The three sides are 4, 4sqrt(3), and 8 The ratio of the sides in a triangle is xxsqrt(3)2x That means if the side opposite 30^circ is x, then the side opposite 60^circ is xsqrt(3) and the side opposite 90^circ is 2x Since the longest side is the hypotenuse, which is opposite 90^circ, we know that 2x = 8\rightarrow x = 4
Question Find the remaining sides of a 30° – 60° – 90° triangle if the longest side is 9 The side opposite 60° is and the shortest side isIt doesn't matter which30 60 90 triangle rules and properties The most important rule to remember is that this special right triangle has one right angle and its sides are in an easytoremember consistent relationship with one another the ratio is a a√3 2a Worksheet 45 ¡45 ¡90 triangleand30 ¡60 ¡90 triangle 1For the 45 ¡45 ¡90 triangle, (the isosceles right triangle), there are two legs of length a and the
The sides of a right triangle lie in the ratio 1√32 The side lengths and angle measurements of a right triangle Credit Public Domain We can see why these relations should hold by plugging in the above values into the Pythagorean theorem a 2 b 2 = c 2 a 2 (a√3) 2 = (2a) 2 a 2 3a 2 = 4a 2 ADVERTISEMENT 4a 2 = 4a 2 Notice that these ratios30°60°90° triangle The 30°60°90° refers to the angle measurements in degrees of this type of special right triangle In this type of right triangle, the sides corresponding to the angles 30°60°90° follow a ratio of 1√ 32 Thus, in this type of triangle, if the length of one side and the side's corresponding angle is known, the length of the other sides can be determinedIn a triangle, the ratio of the sides is always in the ratio of 1√3 2 This is also known as the triangle formula for sides yy√32y Let us learn the derivation of this ratio in the triangle proof section Consider some of the examples of a degree triangle with these side lengths Here, in the triangle DEF ∠ F = 30°, ∠ D = 60°, and




30 60 90 Triangle Theorem Ratio Formula Video



How To Solve 30 60 90 Triangles Krista King Math Online Math Tutor
–find the distance in a 30, 60, 90 triangle 1 answer below » a symmetrical canyon is 4850 feet deep a river runs through the canyon at its deepest point the angle of depression from each side of the canyon to the river is 60o round to theExample of 30 – 60 90 rule Example 1 Find the missing side of the given triangle As it is a right triangle in which the hypotenuse is the double of one of the sides of the triangle Thus, it is called a triangle where smaller angle will be 30 The longer side is always opposite to 60° and the missing side measures 3√3 units inOther interesting properties of triangles are All triangles are similar;




A Triangle With Angle Measures 30 60 90 Has A Perimeter Of 18 6 3 Pwn Test Prep



Pythagorean Triples
Two triangles sharing a long leg form an equilateral triangle;Given the triangle below, find the lengths of the missing sides Since this is a right triangle, we know that the sides exist in the proportion 1\(\sqrt{3}\)2 The shortest side, 1, is opposite the 30 degree angle Since side X is opposite the 60 degree angle, we know that it is equal to \(1*\sqrt{3}\), or about 173Click here👆to get an answer to your question ️ In a 30 60 90 triangle, the length of the hypotenuse is 6 What is the length of the shortest side?




30 60 90 Triangle Explanation Examples
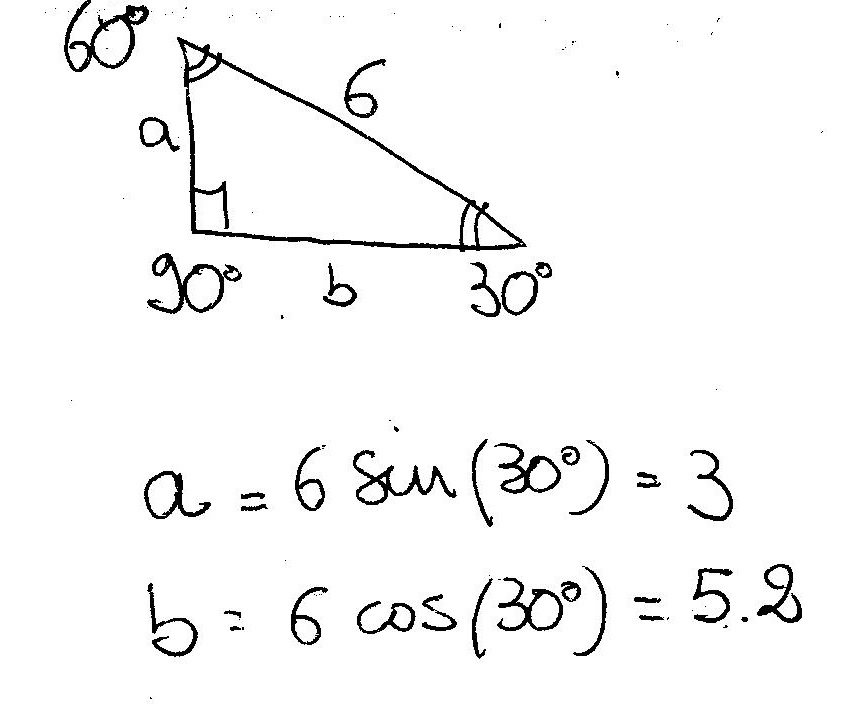



How Do You Find The Remaining Sides Of 30 Circ 60 Circ 90 Circ Triangle If The Longest Side Is 6 Socratic
The triangle is a special right triangle, and knowing it can save you a lot of time on standardized tests like the SAT and ACT Because its angles and side ratios are consistent, test makers love to incorporate this triangle into problems, especially on the nocalculator portion ofAnswer (1 of 3) This question definitely needs to be edited first I guess the question is Q What is the formula to find the hypotenuse in a 30 60 90 triangle If the question is as above FORMULA HYPOTENUSE = √{ s² (√3 s)²}, where s is a side length of the right triangle And obviouAnswer (1 of 3) How do I find the missing sides in special right triangles using the 30–60–90 rule?




30 60 90 Right Triangles Ck 12 Foundation




Relationships Of Sides In 30 60 90 Right Triangles Read Geometry Ck 12 Foundation
How to Solve a Triangle Education is knowing that triangles have three properties laidPractice Using the Triangle to Find Side Lengths with practice problems and explanations Get instant feedback, extra help and stepbyIt turns out that in a triangle, you can find the measure of any of the three sides, simply by knowing the measure of at least one side in the triangle The hypotenuse is equal to twice the length of the shorter leg, which is the side across from the 30 degree angle




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Formula Learn Formula For Calculating The 30 60 90 Measures


